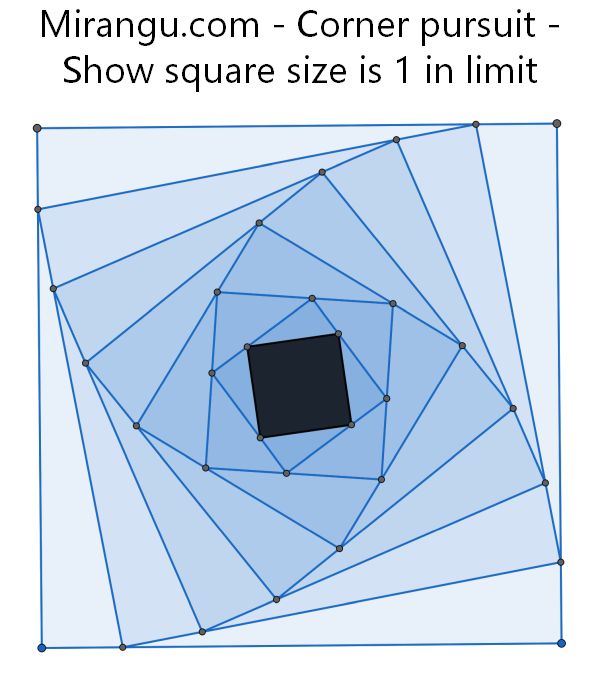
Four corners of a square pursue each other clockwise, and after each unit step mark out the new smaller square. Show that the squares sizes in the sequence decrease in size to 1 in the limit.
Categories
Corner pursuit
Tag: limit
Categories
All-seeing eye
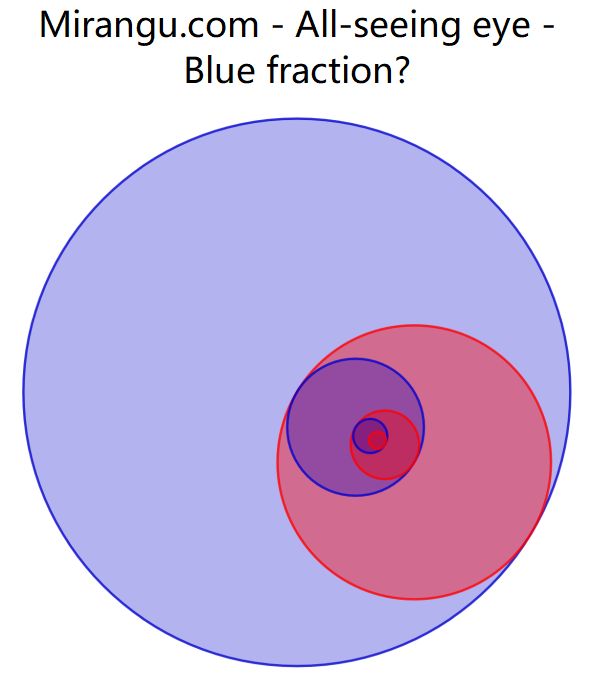
If the radius shrinks by the factor 1/2 for each smaller circle, what fraction of the whole area is represented by all the blue crescents (separated by the red crescents)? Assume the circles keep going inward forever.
Categories
Circle dancing
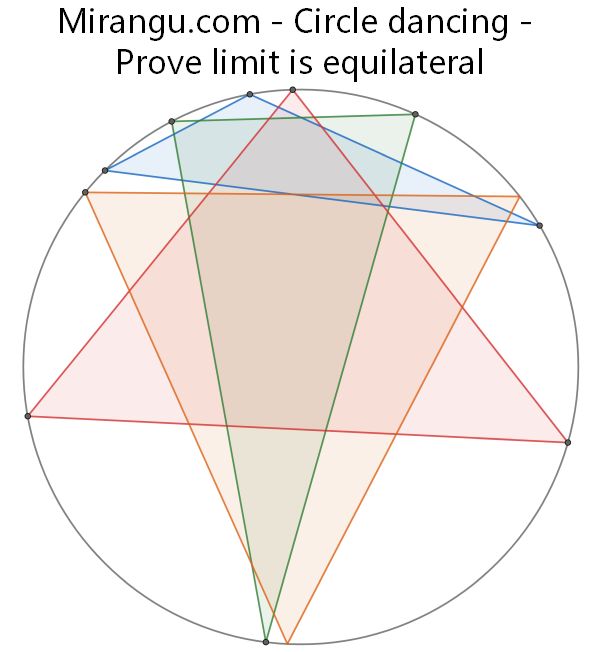
Start with a blue triangle, and form the green triangle whose vertices bisect each circular arc connecting blue vertices. Similarly, make the red triangle from the green, and the orange triangle from the red. Prove the triangle becomes equilateral in the limit.
Categories
The balancing block
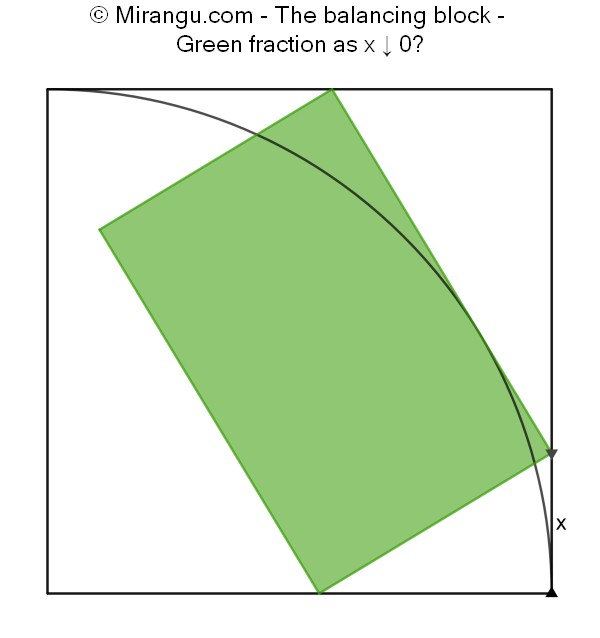
A rectangle inside a square. One of its sides is tangent to the quarter circle. What fraction is green as x approaches 0?
Categories
The rabbit hole
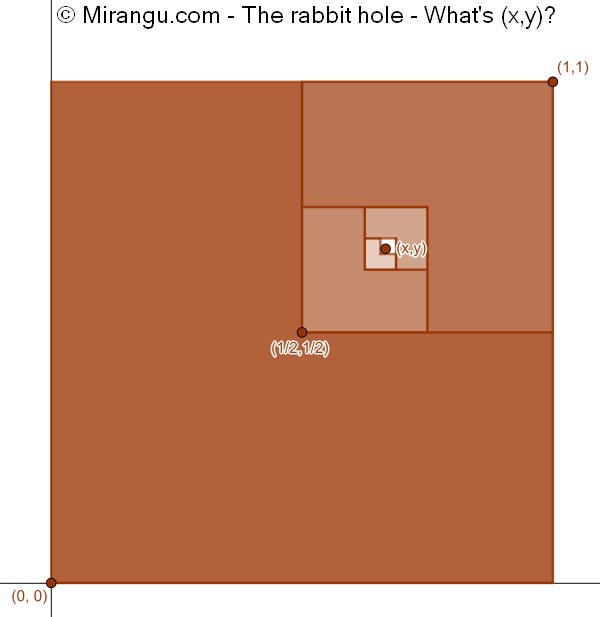
Three quarters of a unit square placed at the origin are shaded as shown. The remaining quarter is treated similarly but mirrored, and so on to infinity. What are the coordinates of the vanishing point?