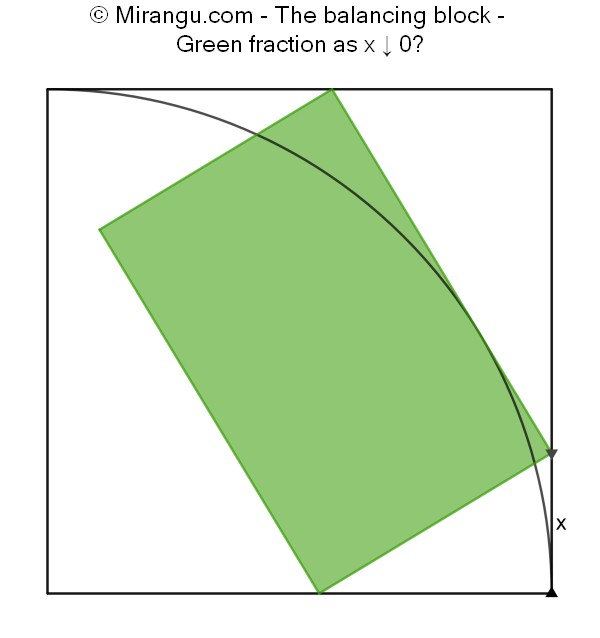
A rectangle inside a square. One of its sides is tangent to the quarter circle. What fraction is green as x approaches 0?

Solution

The fraction in the limit x↓0 is 1/2.

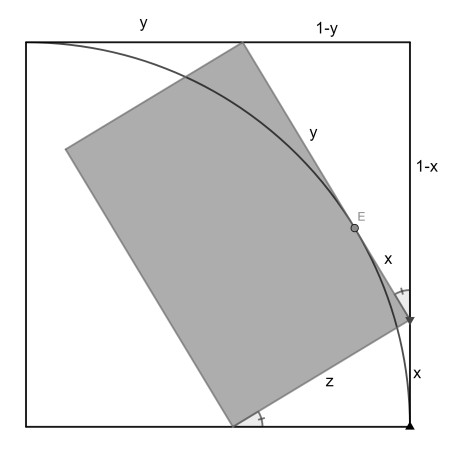
Recognize the three tangency points: upper left and lower right square vertex and point E. From the tangent-tangent theorem we can infer the distances x and y as shown.

Using the Pythagorean theorem in the upper right triangle, we get that y=(1-x)/(1+x), leading to x+y=(1+x²)/(1+x). Using similarity of this triangle and the one in the lower right corner we get z/x=(x+y)/(1-y), leading to z=(1+x²)/2.

Taking the limit x↓0, the long rectangle side tends to 1 and the short one tends to 1/2, leading to a fraction of 1/2.

Poem

A green bloc balances slowly
In a big square closed,
So lonely
The bloc is not free
He wants liberty
And leave this figures so unselfishly
It’s green colour like a flower
Makes him nice and full of power
Who will help and really care?
Maybe you or me, or someone there .

Bella