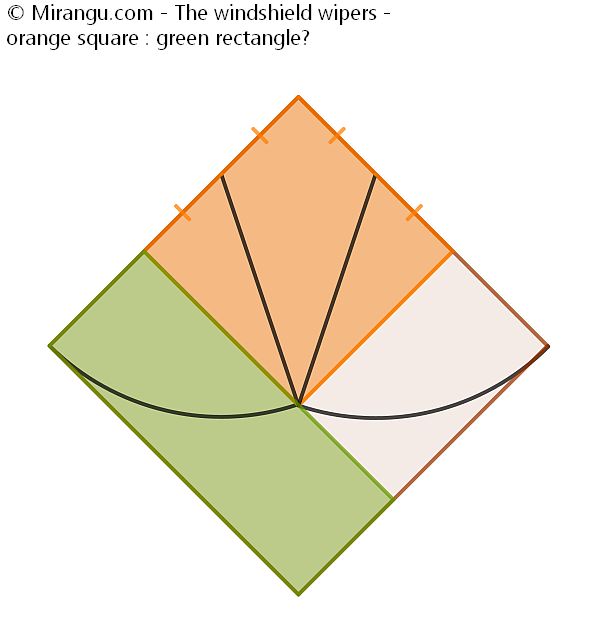
A square and two rectangles, connected by two circular arcs, form a square. What is the proportion of the orange to the green area?
Scroll down for a solution to this problem.
Solution
The green and orange areas have the same size.
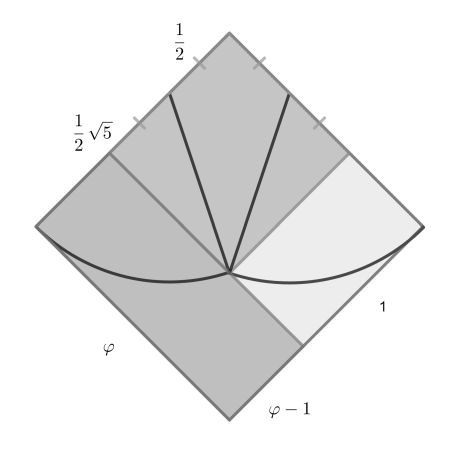
Setting the side of the orange square to 1, it is easily seen from the Pythagorean Theorem that the circular arcs have radius √5/2. Now this makes the large square have side (√5+1)/2, which is the Golden Ratio φ.
The green rectangle has area φ2-φ, which is known to be 1, exactly that of the orange square.