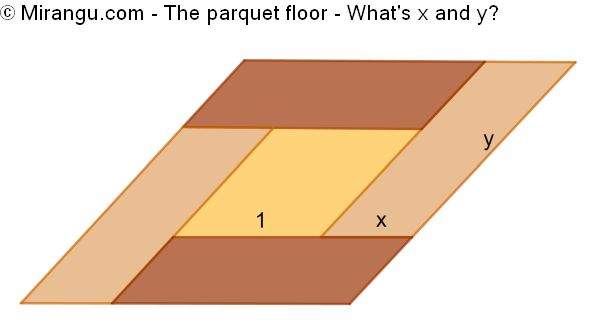
A unit rhombus is surrounded by four congruent parallelograms. All five quadrilaterals have equal area. What are the parallelogram sides x and y?
Scroll down for a solution to this problem.
Solution
The side lengths are x=1/φ and y=φ, where phi is the Golden Ratio (1+√5)/2.
First, the area of a parallelogram is sin(α) times the short side times the long side, where α is either one of the interior angles. In the diagram, this sin(α) term is the same for all parallelograms and can thus be factored out of the equations.
From equating the area of one of the outer paralellologram to the rhombus we get (y-1)y=1. It has one positive root: φ. Also, x=y-1 which is 1/φ.
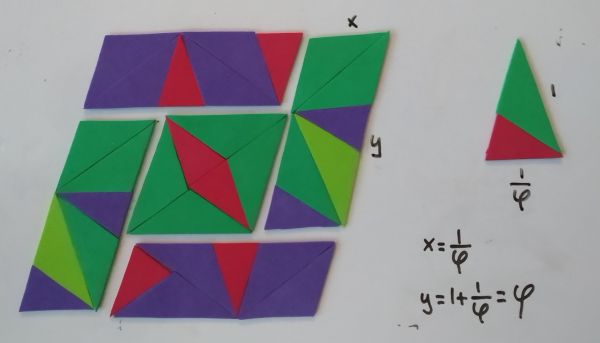
Physical solution
We are very pleased with the solution constructed by Isabel, using physical Golden triangles and gnomons.
Poem
We were honoured to receive the following poem:
C’est le parquet ou encore le sol
Qui nous attire après nos envols
Le parquet où l’on danse
Le parquet de nos transes
Parquet en bois, ciré ou flottant,
Il est figé, immobile ou mouvant,
Le parquet est fixé sur notre terre
Qui nous protège comme une mère nourricière .