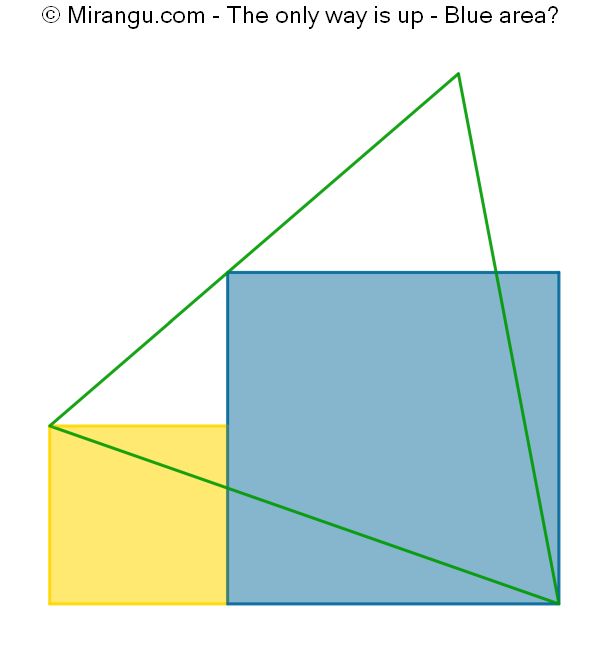
Two squares and an equilateral triangle. If the yellow area is 1, what is the blue area?
Scroll down for a solution to this problem.
Solution
The blue area is 2√3.
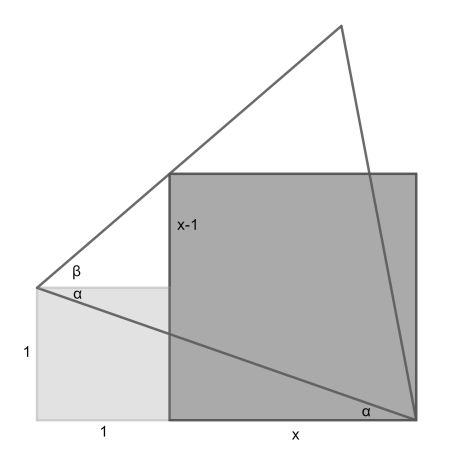
We have added a few lengths and angles in the diagram. Now we can find tan(α)=1/(x+1) and tan(β)=x-1.
Using the trigonometric formula tan(α+β)=(tan(α)+tan(β))/(1-tan(α)tan(β)), we find tan(α+β)=x2/2. Finally, using the fact that α+β=60°, we get x2=2√3.