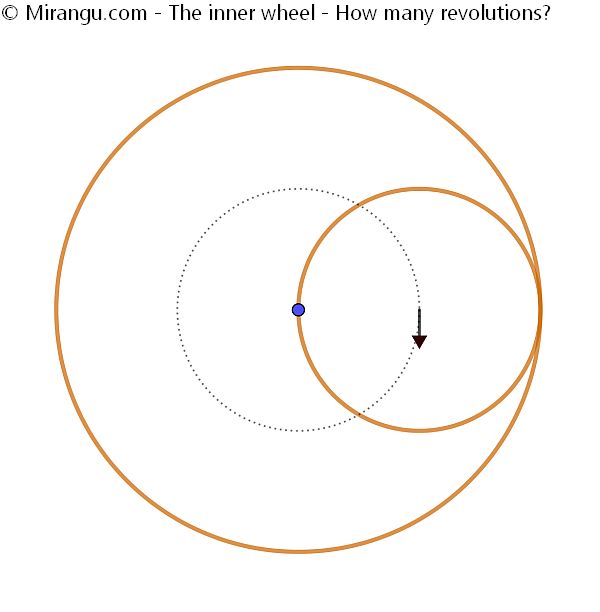
A wheel is placed inside another that has exactly twice its radius. If the inner wheel rolls around once without slipping, how many revolutions has it completed?

Solution

The inner wheel rotates around its axis one time.

If one looks at the proportion of the circumferences of the circles, one would expect an answer of 2 counterclockwise. However, you have to subtract 1 because the inner wheel goes round the circle once clockwise.

This problem is well known for a wheel (or coin) rolling on the outside of another. The inside configuration is known as the Tusi couple. What’s interesting is that a point on the circumference of the inner wheel moves in a straight line along a diameter of the outer wheel.