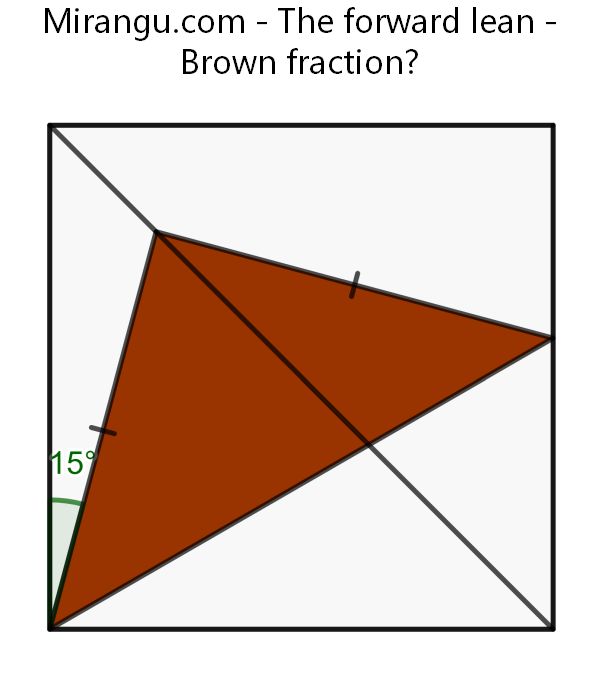
A square with a diagonal and an isosceles triangle. What fraction is brown?
Scroll down for a solution to this problem.
Solution
The brown fraction is 1/3.

First it is easy to see from this diagram that the brown triangle is in fact a right triangle.
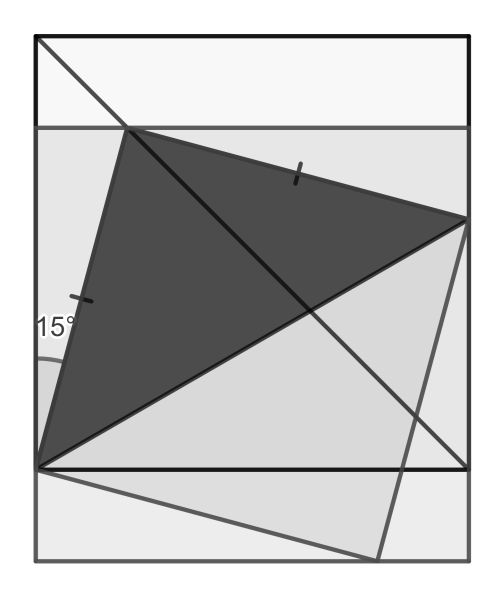
Next we extend the triangle to square and translate the outer square down. The smaller square will fit exactly due to rotational symmetry.
Now setting the smaller side length to a, the four leftover congruent right triangles can be grouped in two isosceles triangles with top angle 30° and equal sides a and therefore having area a2/4. The large square therefore has area 3a2/2, which is three times that of the brown triangle.