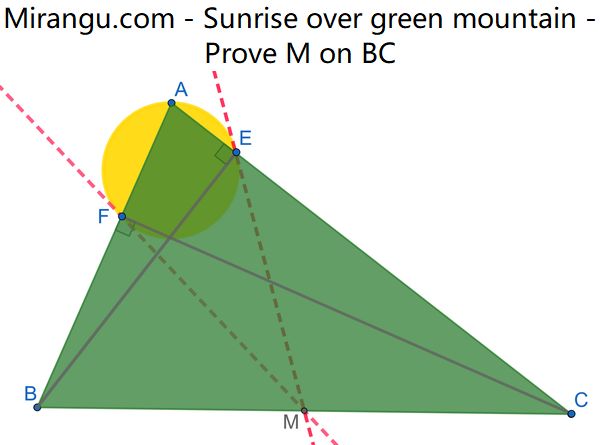
An acute triangle mountain ABC has altitudes BE and CF. The dotted tangent lines to the sunny circumcircle (AEF) at E and F intersect at a point M. Show that M is on the mountain’s base BC.
Scroll down for a solution to this problem.
Solution
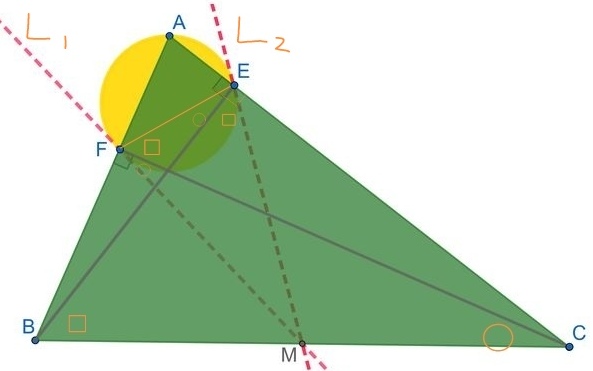
Let L1 and L2 intersect BC at N and O respectively,
∵ ∠CFN=∠FCN,
∴ mid-pt of BC is a pt on L1
∵ ∠BEO=∠EBO,
∴ mid-pt of BC is a pt on L2
∴ mid-pt of BC is interception of L1 and L2 (ie M)
∴ M on BC