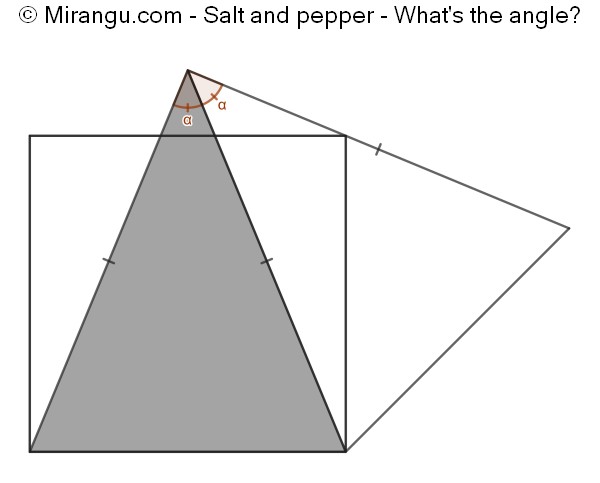
A square and two isosceles triangles. What’s the angle?

Solution

The angle α is 45°.

This solution propagates the angle beta throughout the diagram using:

• Two angles in an isosceles triangle area equal.
• A line cutting two parallel lines forms equal angles.
• The sum of angles on one side of a straight line is 180.

Now we can use the interior angle sum in the small triangle on the top: α+90-β+180-β=180. This gives α=2β-90. We already knew that α=180-2β. Combining the two gives α=45.