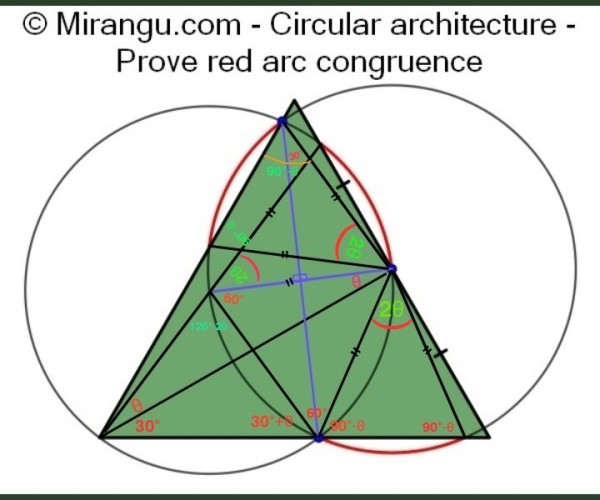
An equilateral triangle and two circles. The centre of the right circle is the triangle side midpoint. Both circle intersections lie on a triangle side. Prove that the three red circular arcs are congruent.
Scroll down for a solution to this problem.
Solution
Poem
Circular architecture
Two moons
Hold a green diamond
Precious jewelry box
Where the tresors are hold
Some cristal pearls
Imagine, rings of gold
They know many secrets
Words for soul and heart
It’s the beauty of the words
Locked in this little jewelery box
A gift for love

