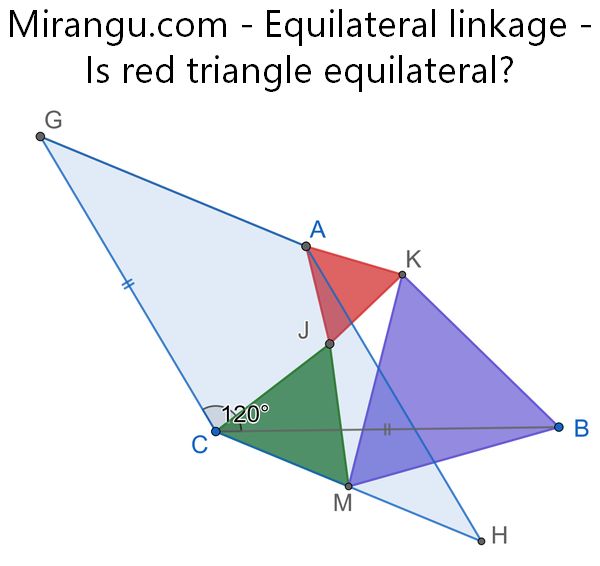
Given 3 points A, B, C, define G so CG=CB and angle GCB is 120 degrees. Define M as the midpoint of side CH of the parallelogram CGAH. The green equilateral has side CM and the purple equilateral has side MB. Is AJK also equilateral?
A square is divided in several triangles and quadrilaterals. What is yellow : red?
A square with several line segments. Prove that the red line segments are parallel.
Two circles with two radii and two tangents. The blue line passes through points of tangency and the circle intersection. Prove that α=β.
Puzzle author: David Odell.
The segment CD is tangent to the green circle c, ending on the orange circle d, which also contains the foot of the perpendicular line from C to the diameter of c which extends to D. What is the angle between the two circles?
A square with two equilateral triangles. What’s the angle α?
The orthocentre H, circumcentre O, the incentre I, and points B and C all lie on the top of the dunce’s spherical head. What is the angle α?
A square and a regular pentagon share a vertex. What’s the angle α?
Two circles are orthogonal (tangents at intersections are perpendicular). How must the points A and B be related?
A square and an equilateral triangle. The square is folded as shown. What is the angle α?